
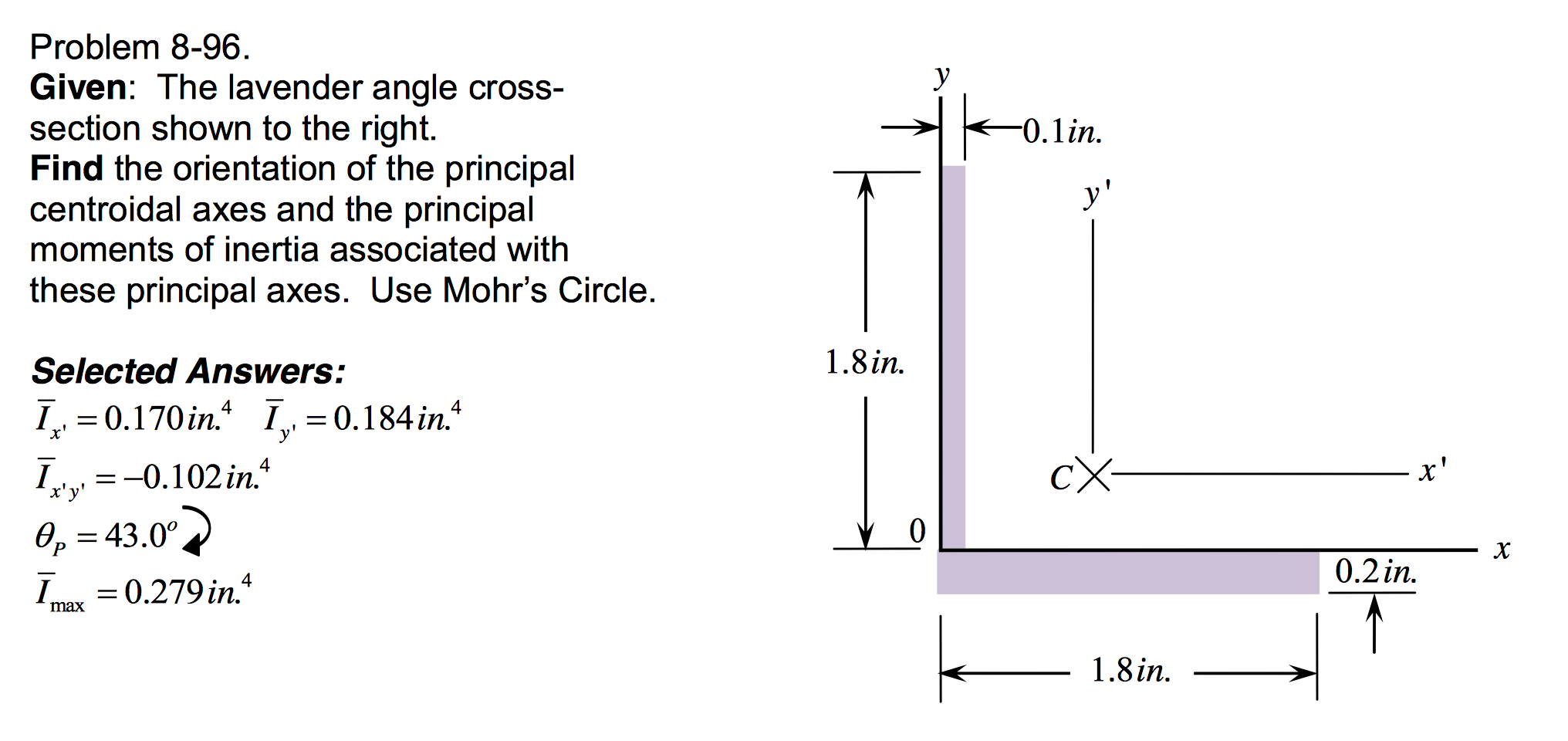
Specifically, the area moment of inertia refers to the second, area, moment integral of a shape, with I xx representing the moment of inertia about the x axis, I yy representing the moment of inertia about the y axis, and J zz (also called the polar moment of inertia) representing the moment of inertia about the z axis. A new axis of rotation ends up with a different formula, even if the physical shape of the object remains the same.Area moments of inertia are used in engineering mechanics courses to determine a bodies resistance to bending loads or torsional loads. The consequence of this formula is that the same object gets a different moment of inertia value, depending on how it is rotating. In this case, the summation over the masses is simply because the two masses at the end of the barbell can be approximated as point masses, and the sum, therefore, has only two terms. To see this, let’s take a simple example of two masses at the end of a massless (negligibly small mass) rod and calculate the moment of inertia about two different axes. How we can find the centre of mass of Earth by using concept of moment of inertia?
The moment of inertia of a uniform semicircular ring of mass M and radius R about the axis perpendicular to plane of ring is 1 ? Using Above Formula You can solve Following types of Question -: Because rr is the distance to the axis of rotation from each piece of mass that makes up the object, the moment of inertia for any object depends on the chosen axis. We defined the moment of inertia I of an object to be for all the point masses that make up the object. For bodies constrained to rotate in a plane, only their moment of inertia about an axis perpendicular to the plane, a scalar value, matters.įor bodies free to rotate in three dimensions, their moments can be described by a symmetric 3 × 3 matrix, with a set of mutually perpendicular principal axes for which this matrix is diagonal and torques around the axes act independently of each other. Its simplest definition is the second moment of mass with respect to distance from an axis. The moment of inertia of a rigid composite system is the sum of the moments of inertia of its component subsystems (all taken about the same axis). It is an extensive (additive) property: for a point mass, the moment of inertia is just the mass times the square of the perpendicular distance to the rotation axis. It depends on the body’s mass distribution and the axis chosen, with larger moments requiring more torque to change the body’s rotation rate. The moment of inertia, otherwise known as the angular mass or rotational inertia, of a rigid body, is a quantity that determines the torque needed for a desired angular acceleration about a rotational axis similar to how mass determines the force needed for the desired acceleration.


 0 kommentar(er)
0 kommentar(er)
